Light that is reflected from the outer surface of the thin film will undergo a
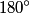 phase shift. Light that is reflected from the inner surface of the thin film will not undergo any phase shift. So, the phase difference between the light reflected from the outer surface of the film and the light reflected from the inner surface of the film will be phase shift. Light that is reflected from the inner surface of the thin film will not undergo any phase shift. So, the phase difference between the light reflected from the outer surface of the film and the light reflected from the inner surface of the film will be where  is the wavelength of the light in the film and is the wavelength of the light in the film and 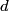 is the thickness of the film. is the thickness of the film.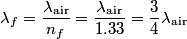 So, 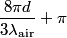 When 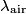 is large enough, the phase shift is approximately is large enough, the phase shift is approximately 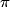 , and there is destructive interference, explaining why the film appears dark. The first wavelength for which the film appears bright corresponds to a phase difference of , and there is destructive interference, explaining why the film appears dark. The first wavelength for which the film appears bright corresponds to a phase difference of 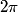 : :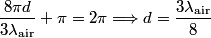 If 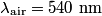 , then , then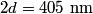 The next wavelength for which the film appears bright corresponds to a phase difference of 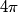 : :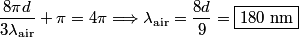 Therefore, answer (B) is correct. |